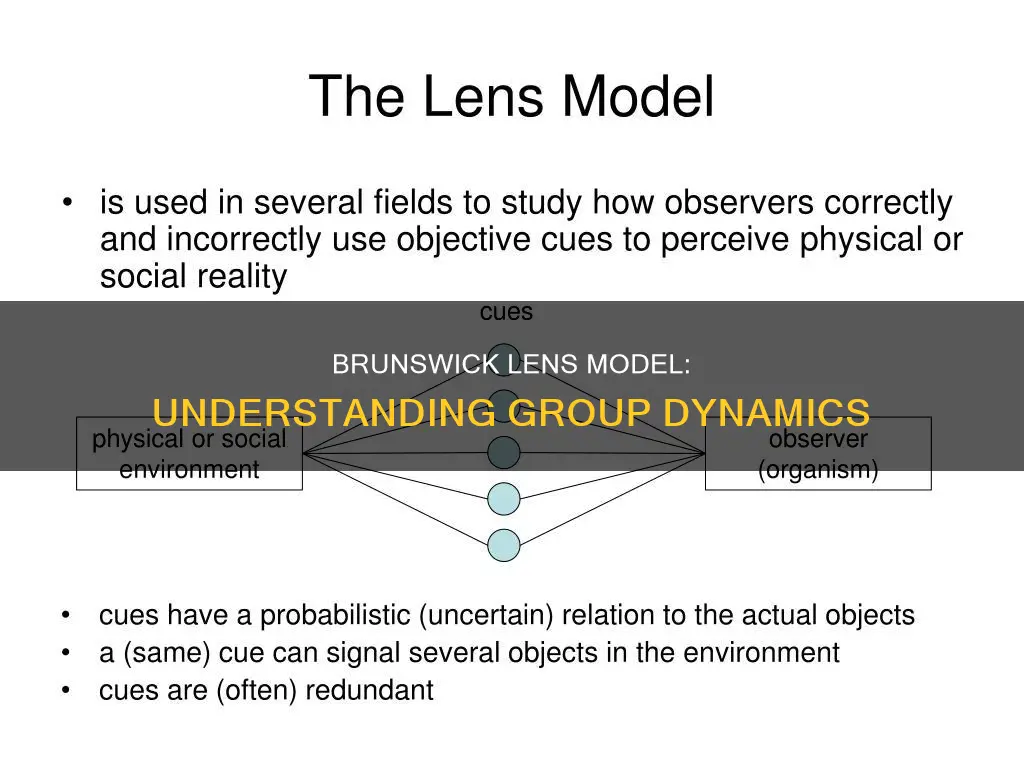
The Brunswik Lens Model, developed by Egon Brunswik, is a conceptual framework for understanding the human decision-making process. It assumes that a decision is composed of three essential elements: the basic information in the situation, the actual decision made, and the optimal or correct decision that should have been made. The model illustrates the interrelationships between these elements, providing insight into the complexity and dynamics of decision-making. It highlights the role of cues or indicators that individuals may use as aids in their decision-making process. These cues can be either valid or invalid, and their utilisation by the decision-maker impacts the quality of their judgement. The Lens Model is a descriptive tool that has been applied in various realistic task settings, offering valuable insights into how people use cues in different situations.
| Characteristics | Values |
|---|---|
| Originator | Egon Brunswik |
| Origin | Brunswik's concept of probabilistic functionalism |
| Purpose | To study the basic characteristics of decision-making |
| Elements | Basic information in the decision situation, the actual decision made, the optimal or correct decision |
| Cues | Indicators that may or may not be used to aid the decision-making process |
| True Cue Validity | The diagnostic or predictive "power" of a cue |
| Observed Cue Validity | The extent to which a decision-maker uses a given cue |
| Decision-Maker Achievement | The correlation between the number of units the executive decided to produce and the number he should have decided to produce |
| Use Case | Understanding the relationships between the environment, attributes, and a person's cognitive system |
What You'll Learn

The Lens Model is a perceptual analogy
The Lens Model, also known as Brunswik's Lens Model, was originally conceived as a perceptual analogy by Egon Brunswik. Brunswik was convinced that the methods of psychological research during the first half of the 20th century were wrong, especially with regard to the "rule of one variable," which many held as the only way to do psychological science. He believed that an essential characteristic of behavior is vicarious functioning, meaning the achievement of organismic goals, either perceptual or instrumental, through a variety of means. The rule of one variable prevents the organism from exercising this fundamental characteristic, thus precluding valid knowledge of behavior.
The Lens Model can be applied to judgment analysis, which requires a substantial number of multi-attribute situations about which a person makes judgments. The purpose of judgment analysis is to understand the relationships between the environment and the attributes (cues), a person's cognitive system and the cues, and the environment and judgment systems.
For example, consider a company interested in the validity of its hiring decisions. Suppose that, for legal reasons, the last 100 applicants have all been hired, regardless of the personnel director's ratings, which were based on five factors. The company has a criterion for success, and the five factors are conceptualized as cues to both judgment and success. Regression analysis can be used to assess the contribution of the cues to successful prediction and the relationship of the cues to success on the job. The Lens Model provides a rich tool for understanding the relationships between the person and the environment in this context.
The Lens Model has been applied in various fields, including industrial and organizational psychology. It is a valuable tool for understanding the interrelations between people and their environment and has been used in tasks such as performance assessment, employment interviewing, investment decisions, evaluation of discrimination charges, and assessment of the desirability of employment contracts.
Exploring the East Coast: Driving to PEI
You may want to see also

The model assumes three essential elements
The Brunswik Lens Model assumes three essential elements:
Basic Information in the Decision Situation
The first element of the model is the basic information available to the decision-maker. This includes all the potential cues or indicators that can be used to inform the decision. For example, an executive deciding on the monthly production quantity of a product might consider the present inventory, current orders, market indicators, and advice from subordinates. These cues provide information that can help guide the decision-making process.
Actual Decision Made by the Decision-Maker
The second element is the actual decision that is made. This involves a choice of action, or in some cases, the decision not to act. In the example of the executive, the decision would be the number of units of the product to produce for the month. This decision is the observable outcome of the decision-making process and represents the course of action to which the decision-maker commits.
Optimal or Correct Decision
The third element is the optimal or correct decision that should have been made in that particular situation. This represents the best possible choice of action that could have been selected. It serves as the criterion against which the actual decision should be evaluated. However, it is important to note that determining the optimal decision can be challenging and may not always be clear, especially in complex or uncertain situations.
The model examines the interrelationships between these three elements, providing insight into the complexity and dynamics of the decision-making process. It helps to assess the quality of the decision by evaluating how well the decision-maker utilised the available information and how close their decision was to the optimal choice.
The Brunswik Lens Model is a descriptive conceptualisation of the human decision-making process, offering a framework to study and understand how individuals make choices and the factors that influence their decisions.
Rutgers New Brunswick: Architecture Programs?
You may want to see also

True and Observed Cue Validity
The Brunswik Lens Model, developed by Egon Brunswik in 1956, is a conceptual framework for understanding the decision-making process. The model assumes that the decision-making process is composed of three essential elements: basic information, the actual decision, and the optimal decision.
True cue validity refers to the correlation between a cue and the correct decision, indicating how helpful a particular cue is in the decision-making process. In other words, it represents the diagnostic or predictive power of a cue. For example, consider an executive who needs to decide on the monthly production quantity of a product. One cue they might use is the size of their present inventory. By looking at past records, they can determine the correlation between inventory size and the optimal number of units to produce. A strong negative correlation would indicate that low inventory values correspond to a high number of units needed, making inventory size a valuable cue.
Observed cue validity, on the other hand, addresses the question of how well a decision-maker uses a given cue. This is determined by examining the correlation between the cue values and the decisions made over time. Continuing with the previous example, if the executive's decisions to produce a certain number of units are only slightly correlated with the inventory size, it suggests that they are not fully utilizing this valuable cue.
The Brunswik Lens Model helps identify the interrelationships between the essential elements of the decision-making process, providing insights into its complexity and dynamics. It also offers mathematical indices for studying human decision-making and has been applied in various research studies to understand how people use cues in different situations.
Fixing Brunswick Pool Table Pockets
You may want to see also

Decision-Maker Achievement
The Brunswick Lens Model is a probabilistic approach to decision-making, mapping a finite set of cues onto a decision object through a weighting scheme. The Lens Model assumes that the decision process is composed of three essential elements: the basic information in the decision situation, the actual decision made by the decision maker, and the optimal or correct decision that should have been made in that particular situation.
Research based on the Lens Model has yielded several interesting findings about the ability of people to use cues in decision-making. For instance, studies have shown that decision-makers can learn to use cues appropriately, giving more attention to good cues than poor ones. However, an interesting finding by Dudycha and Naylor suggests that providing a decision-maker with a second, poorer cue that still has some predictive value after they already have a very good cue will decrease their performance. Apparently, poor cues add more noise to the decision-making process than predictive value.
Another finding, reported by Clark, indicates that cues with negative validity are not as useful to decision-makers as those with a direct or positive relationship. Humans seem to have more difficulty learning to use information sources that give negative validity, even though the predictive value of a cue is not affected by whether the relationship is positive or negative.
Other findings include the fact that humans are better at learning to use cues with linear relationships to the correct decision than those with nonlinear relationships, and that humans tend to use cues systematically even when they have no predictive power.
Gift Certificates: Validity in New Brunswick
You may want to see also

The Lens Model Equation
> \(\frac { 1 } { \mathrm { d } _ { \mathrm { o } } } + \frac { 1 } { \mathrm { d } _ { \mathrm { i } } } = \frac { 1 } { \mathrm { f } }\).
This equation can be derived from a geometric analysis of ray tracing for thin lenses. Here's a breakdown of the components:
- Object Distance (do): This refers to the distance of an object from the centre of a lens. It is always a positive value, unless multiple lenses are used.
- Image Distance (di): This is the distance of the image from the centre of the lens. The image distance can be positive or negative, depending on its position relative to the lens and the observer's eye. If the image is on the opposite side of the lens from the observer's eye, it is considered positive.
- Focal Length (f): The focal length is the distance from the centre of the lens to its focal point. For a convex lens (also known as a converging lens), the focal length is always positive. For a concave lens (also known as a diverging lens), the focal length is always negative.
The magnification of an image can be calculated using the following formula:
> \(\frac { \mathrm { h } _ { \mathrm { i } } } { \mathrm { h } _ { \mathrm { o } } } = - \frac { \mathrm { d } _ { \mathrm { i } } } { \mathrm { d } _ { \mathrm { o } } } = \mathrm { m }\).
Here, hi and ho represent the heights of the image and object, respectively. The magnification (m) provides information about the size and orientation of the image. A positive magnification indicates a right-side-up image, while a negative magnification means the image is inverted.
Brunswick to Maryland: Travel Time
You may want to see also
Frequently asked questions
The Brunswik Lens Model is a conceptual framework for viewing and understanding the decision-making process. It assumes that any decision is composed of three essential elements: the basic information in the decision situation, the actual decision made, and the optimal or correct decision that should have been made.
The key elements of the model are the decision variables or "cues" available to the decision-maker, the observed course of action taken, and the optimal response or "correct" decision. These elements are interrelated and provide insight into the complexity and dynamics of the decision-making process.
The model analyses true cue validity and observed cue validity. True cue validity refers to the diagnostic or predictive power of a cue, indicating how helpful it is in the decision-making process. Observed cue validity, on the other hand, assesses the extent to which the decision-maker utilised a given cue by examining the correlation between the cue values and the actual decision made.
The model is used in judgment analysis to understand the relationships between the environment and its attributes (cues), the decision-maker's cognitive system, and the environment and judgment systems. It helps evaluate the validity of judgments and predictions made by individuals or groups, providing insight into the interrelations between people and their environment.